progressiveoreo.blogg.se

1. #Series and sequences math and science initiative how to#
2. #Series and sequences math and science initiative code#
3. #Series and sequences math and science initiative series#

It's true that the Fibonacci sequence is tightly connected to what's now known as the golden ratio, phi, an irrational number that has a great deal of its own dubious lore.

#Series and sequences math and science initiative code#

However, it's not some secret code that governs the architecture of the universe, Devlin said. Other than being a neat teaching tool, the Fibonacci sequence shows up in a few places in nature. (Image credit: Shutterstock) Why is the Fibonacci sequence important? The Fibonacci sequence and the golden ratio are eloquent equations, but they aren't as magical as they may seem. In 1877, French mathematician Édouard Lucas officially named the rabbit problem "the Fibonacci sequence," Devlin said. In fact, it was mostly forgotten until the 19th century, when mathematicians worked out more about the sequence's mathematical properties. But after a few scant paragraphs on breeding rabbits, Leonardo of Pisa never mentioned the sequence again. "Liber Abaci" first introduced the sequence to the Western world. Read more: 9 equations that changed the world The answer, it turns out, is 144 - and the formula used to get to that answer is what's now known as the Fibonacci sequence. (Ignore the wildly improbable biology here.) After a year, how many rabbits would you have? A month later, those rabbits reproduce and out comes - you guessed it - another male and female, who also can mate after a month. After a month, they mature and produce a litter with another male and female rabbit. The problem goes as follows: Start with a male and a female rabbit. In one place in the book, Leonardo of Pisa introduces the sequence with a problem involving rabbits. Written for tradesmen, "Liber Abaci" laid out Hindu-Arabic arithmetic useful for tracking profits, losses, remaining loan balances and so on, he added.

#Series and sequences math and science initiative how to#

However, in 1202 Leonardo of Pisa published the massive tome "Liber Abaci," a mathematics "cookbook for how to do calculations," Devlin said. (Image credit: Stefano Bianchetti/Corbis via Getty Images) However, in 1202 in a massive tome, he introduces the sequence with a problem involving rabbits.

To know which ones to use, look for the keyword Arithmetic or Geometric in the question.Portrait of Leonardo Fibonacci, who was thought to have discovered the famous Fibonacci sequence. Knowing a and r we can find the value of any term or the sum of the first n terms using the formulae below.Īll these formulas are found on page 22 of the “Formulae and Tables” booklet which will be provided during the Inter Cert and Leaving Cert exams. Knowing a and d we can find the value of any term or the sum of the first n terms using the formulae below.Ī pattern of numbers obtained by multiplying any term by a common ratio to get the next number in the pattern is known as a Geometic Sequences or Series.

#Series and sequences math and science initiative series#

S n is represents the sum of the first n numbers in a pattern.Īrithmetic Sequences and Series (also called linear)Ī pattern of numbers obtained by adding a common difference to each entry to get the next one is known as an Arithmetic or Linear Sequences or Series. The letter “r” is used to represent the common ratio or number by which each term is multiplied to get the next one. It is the common difference between adjacent numbers. The letter “d” is used for a constant number that is added to each number in a pattern to get the next one. The first number in a pattern of numbers is often designated “a” in formulae and could also be designated T 1. The term number is written as a subscript under the T. But the algebra content is usually nowhere near that required in algebra itself, functions and differentiation.Ī sequence is an ordered list of numbers and the sum of the terms of a sequence is a series. Like most other questions on the first paper, a certain proficiency with algebra is required to answer many of the harder questions on sequences and series. Sequences and series are examined in Question 5 on Paper 1 each year. However, we only have to investigate two kinds on our course, namely arithmetic and geometric sequences and series. Also, there are many different kinds of sequence and series. Like most ideas treated mathematically, we find that we have to introduce terminology and notation to allow us to develop fully the properties of series. So what is a series? A series is just what we get when we add some of the terms of a sequence, e.g., 1+4+9+16. In the example above, the next number is 25, because it is the square of 5. In practice, the numbers in the list usually follow some pattern. The list of numbers, separated by commas, is a sequence.

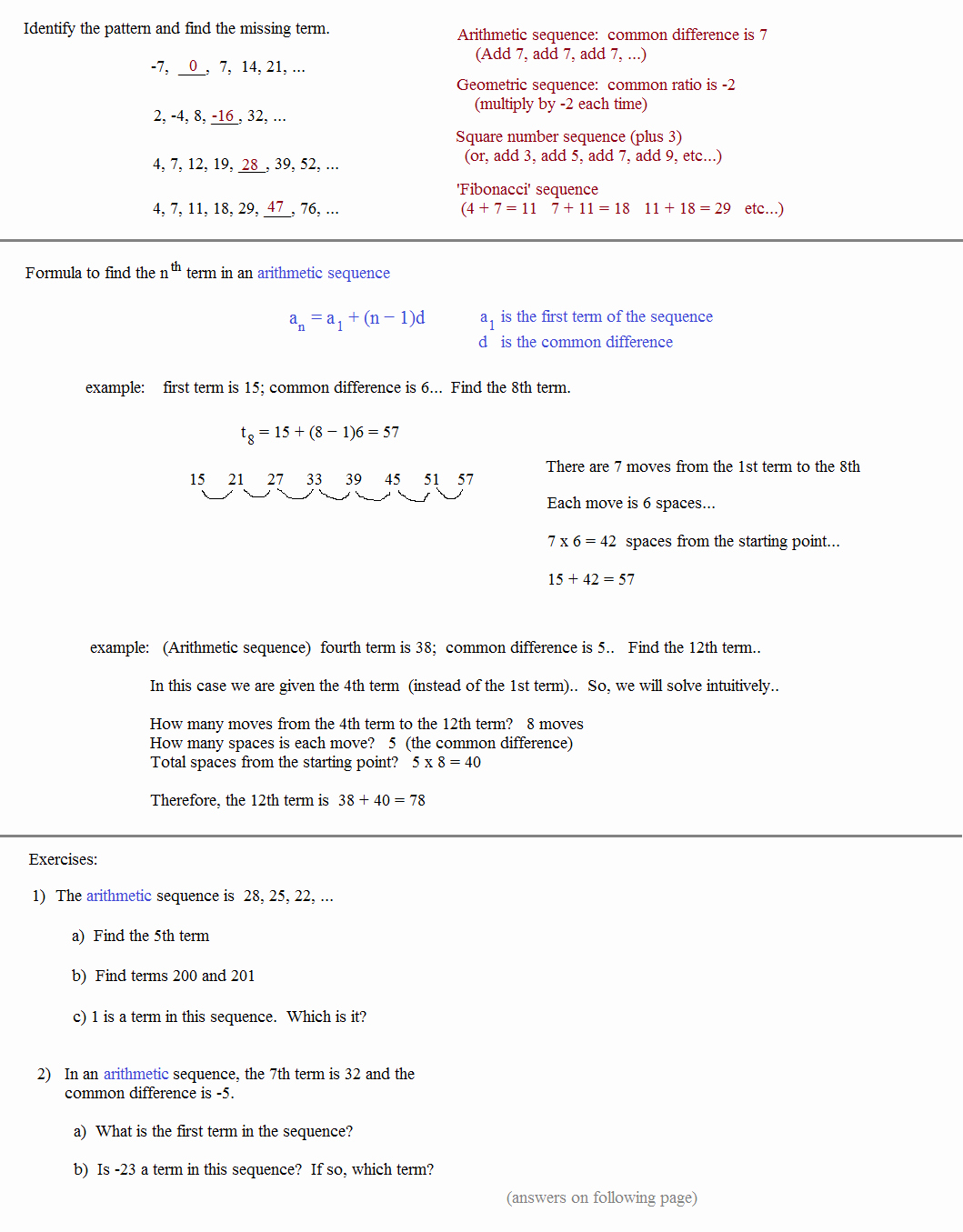
Questions of the form “what is the next number in the list 1, 4, 9, 16, …” frequently appear in quizzes. Most people have met sequences at some stage in their lives.